Diagonalfunktor

Im mathematischen Teilgebiet der Kategorientheorie ist der Diagonalfunktor ein Funktor, der es erlaubt, eine Kategorie ${\displaystyle {\mathcal {C}}}$ in die Kategorie der Funktoren ${\displaystyle {\mathcal {C}}^{\mathcal {D}}}$ für eine beliebige nichtleere (kleine) Kategorie ${\displaystyle {\mathcal {D}}}$ einzubetten. Der Name rührt daher, dass für ein diskretes ${\displaystyle {\mathcal {D}}}$ mit zwei Elementen der Diagonalfunktor gerade die Abbildung ${\displaystyle {\mathcal {C}}\to {\mathcal {C}}\times {\mathcal {C}},u\mapsto (u,u)}$ ist.

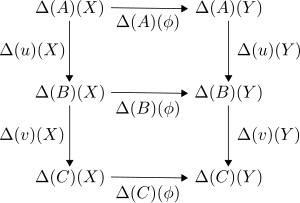
Sei ${\displaystyle {\mathcal {C}}}$ eine Kategorie und ${\displaystyle {\mathcal {D}}}$ eine kleine Kategorie. Dann ist der Diagonalfunktor ${\displaystyle \Delta }$ definiert als Abbildung, die jedem Morphismus ${\displaystyle u\in {\mathcal {C}}}$ eine natürliche Transformation ${\displaystyle \Delta (u)\in {\mathcal {C}}^{\mathcal {D}}}$ zuordnet, wobei ${\displaystyle \Delta (u)}$ dadurch gegeben sei, dass sie jedem Objekt und damit jedem Morphismus in ${\displaystyle {\mathcal {D}}}$ den Morphismus ${\displaystyle u}$ zuweise. Für ein Objekt ${\displaystyle A\in {\mathcal {C}}}$ ist ${\displaystyle \Delta (A)}$ offensichtlich ein Funktor. Um nun einzusehen, dass ${\displaystyle \Delta }$ tatsächlich Funktor ist, betrachte man für Morphismen ${\displaystyle u\colon A\to B}$ und ${\displaystyle v\colon B\to C}$ aus der Kategorie ${\displaystyle {\mathcal {C}}}$ die Verkettung der natürlichen Transformationen ${\displaystyle \Delta (u)}$ und ${\displaystyle \Delta (v)}$, dies ergibt per Definition für jedes ${\displaystyle \phi \colon X\to Y}$ in ${\displaystyle {\mathcal {D}}}$ das folgende kommutative Diagramm:

Dieses ist nichts anderes als:

Dies entspricht der natürlichen Transformation ${\displaystyle \Delta (uv)}$, womit bewiesen ist, dass ${\displaystyle \Delta (uv)=\Delta (u)\Delta (v)}$. Für nichtleeres ${\displaystyle {\mathcal {D}}}$ ist ${\displaystyle \Delta }$ offensichtlich injektiv, bettet also ${\displaystyle {\mathcal {C}}}$ in die entsprechende Funktorkategorie ein. Unter einer bestimmten Voraussetzung ist ${\displaystyle \Delta }$ auch voll: Sei ${\displaystyle \alpha \colon \Delta (A)\to \Delta (B)}$ natürliche Transformation, d. h., dass für jedes ${\displaystyle \phi \colon X\to Y}$ in ${\displaystyle {\mathcal {D}}}$ das Diagramm

kommutiert (denn ${\displaystyle \Delta (A)(\phi )=A}$ und ${\displaystyle \Delta (B)(\phi )=B}$). Was nichts anderes heißt, als dass ${\displaystyle \alpha (X)=\alpha (Y)}$, wann immer ein Morphismus zwischen ${\displaystyle X}$ und ${\displaystyle Y}$ existiert. Falls die Kategorie ${\displaystyle {\mathcal {D}}}$ als Graph aufgefasst schwach zusammenhängend ist, ist ${\displaystyle \alpha }$ also konstant und somit im Bild von ${\displaystyle \Delta }$, womit ${\displaystyle \Delta }$ voll ist.^[1] Dies ist beispielsweise für eine Pfeilkategorie ${\displaystyle {\mathcal {C}}^{\mathcal {D}}}$ oder allgemeiner für ${\displaystyle {\mathcal {D}}}$ mit Anfangs- oder Endobjekt erfüllt, nicht dagegen für ein Produkt ${\displaystyle {\mathcal {C}}^{\mathcal {D}}}$ für diskretes ${\displaystyle {\mathcal {D}}}$ mit mindestens zwei Elementen.

Ein Kegel bezüglich eines Funktors ${\displaystyle F\colon {\mathcal {D}}\to {\mathcal {C}}}$ ist nichts anderes als ein Objekt in ${\displaystyle {\mathcal {C}}}$ versehen mit einer natürlichen Transformation von ${\displaystyle \Delta (A)}$ nach ${\displaystyle F}$. Ein Limes von ${\displaystyle F}$ ist dabei ein spezieller Kegel, nämlich eine ${\displaystyle \Delta }$-kouniverselle Lösung für ${\displaystyle F}$. Dual dazu ist ein Kolimes von ${\displaystyle F}$ ein spezieller Kokegel, nämlich eine ${\displaystyle \Delta }$-universelle Lösung für ${\displaystyle F}$. Besitzt ${\displaystyle \Delta }$ einen rechtsadjungierten Funktor, so ist ${\displaystyle {\mathcal {C}}}$ vollständig bezüglich Limites auf ${\displaystyle {\mathcal {D}}}$, die Umkehrung gilt ebenfalls. Dieser adjungierte Funktor ist gerade der Limesfunktor. Entsprechend ist der Kolimesfunktor (wenn er existiert) linksadjungiert zum Diagonalfunktor.^[2]

Der Diagonalfunktor ist stetig, d. h., er erhält alle Limites, die in ${\displaystyle {\mathcal {C}}}$ existieren. Ebenso erhält er alle Kolimites.^[3]

• Saunders Mac Lane: Categories for the Working Mathematician. 2. Auflage. Springer, New York 1998, ISBN 0-387-98403-8. 
• Dieter Pumplün: Elemente der Kategorientheorie. 1. Auflage. Spektrum Akademischer Verlag, Heidelberg 1999, ISBN 3-86025-676-9. 

• diagonal functor, Eintrag im nLab. (englisch)

1. ↑ Pumplün, S. 105–106
2. ↑ Mac Lane, S. 233
3. ↑ Pumplün, S. 169